Directrix And Focus of a Parabola - Definition, Properties,Examples

Author：Ferdinand University teacher - Tutor for 7 years

The directrix and focus are fundamental elements in defining a parabola, a type of conic section or curve. Each parabola is symmetrical and has a single axis of symmetry. The focus and directrix work together to provide a unique way to construct or understand the shape and orientation of a parabola.

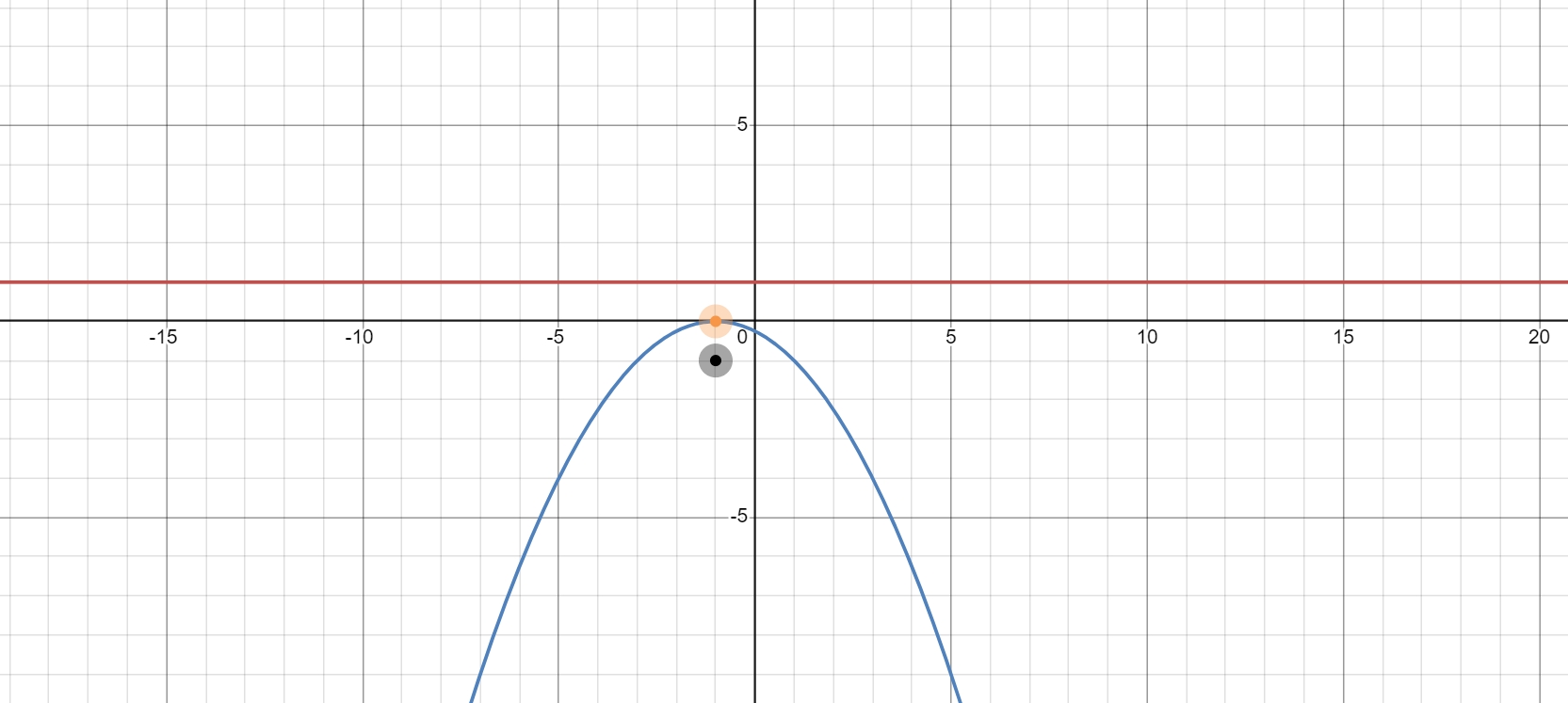
Focus:

The focus of a parabola is a fixed point located inside the curve. It has a unique property: for any point on the parabola, the distance to the focus is equal to the distance to the directrix (a straight line). The focus lies on the axis of symmetry of the parabola.

Directrix:

The directrix of a parabola is a line that is perpendicular to the axis of symmetry of the parabola and does not intersect the parabola itself. The distance between any point on the parabola and the directrix is equal to the distance between that point and the focus. This constant distance defines the parabola's curvature and width.

Relationship and Properties:

• Equidistance property: Every point on the parabola is equidistant from the focus and the directrix. This property is fundamental to the mathematical definition of a parabola.
• Formula and Orientation: The standard form of a parabola's equation depends on its orientation (vertical or horizontal) and its distance from the focus to the vertex (point where the parabola changes direction).

For a vertical parabola with vertex at the origin, the equation can be written as $y = ax^2$ if the focus is above the directrix, and $y = -ax^2$ if the focus is below the directrix, where $4a$ is the distance between the vertex and the focus (or the vertex and the directrix). For a horizontal parabola, the roles of $x$ and $y$ are switched.

How to find the directrix and focus of a parabola?

Finding the directrix and focus of a parabola involves using the equation of the parabola and its geometric properties. Here's how to do it step by step:

Given Equation Form:

Assume the parabola has a vertex at the origin $(0, 0)$ and is oriented either vertically (opening upwards or downwards) or horizontally (opening to the right or left). The standard equations are:

• For a vertical parabola: $y = ax^2$
• For a horizontal parabola: $x = ay^2$

where $a$ is a constant that determines the "openness" of the parabola.

Step 1: Identify the Orientation and Equation

First, determine if the parabola is vertical ($y = ax^2$) or horizontal ($x = ay^2$). This will tell you the direction in which the parabola opens.

Step 2: Determine the Value of $a$

The value of $a$ in the equation tells you how "wide" or "narrow" the parabola is and helps in finding the focus and directrix.

Finding the Focus:

• For a vertical parabola $y = ax^2$, the focus is at $(0, \frac{1}{4a})$.
• For a horizontal parabola $x = ay^2$, the focus is at $(\frac{1}{4a}, 0)$.

Finding the Directrix:

• For a vertical parabola $y = ax^2$, the directrix is a horizontal line given by $y = -\frac{1}{4a}$.
• For a horizontal parabola $x = ay^2$, the directrix is a vertical line given by $x = -\frac{1}{4a}$.

Solved Example

Examples for a Vertical Parabola:

Given: $y = 4x^2$

Find $a$:

Here, $a = 4$, so the coefficient $1/4a = 1/16$.

Focus: The focus is $(0, 1/16)$.

Directrix: The directrix is the line $y = -1/16$.

Example for a Horizontal Parabola:

Given: $x = \frac{1}{2}y^2$

Find $a$:

Here, $a = 1/2$, so $1/4a = 1/2$.

Focus:

The focus is $(1/2, 0)$.

Directrix:

The directrix is the line $x = -1/2$.

Parabola equation from focus and directrix

To derive the equation of a parabola from its focus and directrix, you can use the definition of a parabola: it is the set of all points in a plane that are equidistant from a fixed point (the focus) and a fixed line (the directrix).

Given:

• Focus: $F(p, q)$
• Directrix: Line with equation $ax + by + c = 0$

Objective:

Derive the equation of the parabola.

Steps:

Step 1: Use the Distance Formula

For any point $P(x, y)$ on the parabola, the distance to the focus $F(p, q)$ is equal to the perpendicular distance from $P(x, y)$ to the directrix $ax + by + c = 0$.

Distance to Focus:

$D_F = \sqrt{(x - p)^2 + (y - q)^2}$

Distance to Directrix:

$D_D = \frac{|ax + by + c|}{\sqrt{a^2 + b^2}}$

Step 2: Set Distances Equal

Because $D_F = D_D$, you have: $\sqrt{(x - p)^2 + (y - q)^2} = \frac{|ax + by + c|}{\sqrt{a^2 + b^2}}$

Squaring both sides to eliminate the square root gives: $(x - p)^2 + (y - q)^2 = \left(\frac{ax + by + c}{\sqrt{a^2 + b^2}}\right)^2$

Step 3: Simplify the Equation

Depending on the orientation and specific values of $p$, $q$, $a$, $b$, and $c$, you'll expand and simplify the equation to find the standard form of the parabola.

Example for a Vertical Parabola:

Given:

• Focus: $F(0, p)$ (assuming $p > 0$)
• Directrix: $y = -p$

Using the definition:

Distance to Focus:

For any point $P(x, y)$ on the parabola, the distance to the focus is: $D_F = \sqrt{(x - 0)^2 + (y - p)^2}$

Distance to Directrix:

The distance to the directrix $y = -p$ is simply: $D_D = |y + p|$

Setting $D_F = D_D$ and squaring both sides, we get: $x^2 + (y - p)^2 = (y + p)^2$

Expanding and then simplifying: $x^2 + y^2 - 2yp + p^2 = y^2 + 2yp + p^2$ $x^2 = 4yp$

Since the formula for a vertical parabola with vertex at the origin can be written as $y = \frac{1}{4p}x^2$, we match the derived equation with this form: $y = \frac{1}{4p}x^2$

FAQs about The directrix and focus Of Parabola

Q: What is the general equation of a parabola?

A: The general equation of a parabola depends on its orientation (vertical or horizontal) and whether the vertex is at the origin or another point. Here are the standard forms:

Parabola with Vertex at the Origin:

1. Vertical Orientation (opening upwards or downwards):

   • Equation: (y = ax^2)
     • Here, (a) determines the "width" and the direction of the opening of the parabola. If (a > 0), the parabola opens upwards. If (a < 0), it opens downwards.

2. Horizontal Orientation (opening to the right or left):

   • Equation: (x = ay^2)
     • In this case, (a) also affects the "width" and direction. If (a > 0), the parabola opens to the right. If (a < 0), it opens to the left.

Parabola with Vertex at Any Point ((h, k)):

1. Vertical Orientation:

   • Equation: ((y - k) = a(x - h)^2)
     • This is a translation of the parabola (y = ax^2) to the point ((h, k)), preserving its "width" and direction of opening.

2. Horizontal Orientation:

   • Equation: ((x - h) = a(y - k)^2)
     • Similarly, this translates the parabola (x = ay^2) to the point ((h, k)), maintaining its characteristics.

Q: What is the general equation of a parabola?

A: Parabolas, due to their unique geometrical properties, find extensive applications in various real-life situations and fields. Here are some prominent examples:

1. Engineering and Physics:

• Satellite Dishes and Telescopes: These devices often utilize a parabolic reflector to focus signals or light to a single point, the focus, enhancing signal strength or image clarity.
• Suspension Bridges: The cables of a suspension bridge form a parabolic shape due to uniform gravitational force acting on them, helping in the distribution of tension.

2. Sports:

• Projectile Motion: The path of a ball thrown or an arrow shot follows a parabolic trajectory when air resistance is negligible. This principle helps athletes in sports like basketball, soccer, or archery to aim.

3. Architecture and Design:

• Parabolic Arches: Used in structures for their strength and aesthetic appeal. The Gateway Arch in St. Louis is a famous example of a parabolic arch.

4. Automotive Headlights:

• Focusing Light: The parabolic shape of car headlights helps in focusing the light beam into a parallel beam, improving visibility.

5. Acoustics:

• Sound Amplification: Parabolic microphones and speakers can focus sound waves to a point, allowing for sound amplification and selective hearing over long distances.

6. Astronomy:

• Telescopes: Many optical telescopes use parabolic mirrors to collect and focus light from distant stars and galaxies, making them visible or photographable.

7. Photography:

• Parabolic Reflectors: Used in photography and filming to focus light for better illumination of a subject or scene.

8. Water Fountains:

• Path of Water: The path of water streams in many fountains tends to follow a parabolic trajectory, which is manipulated for creating pleasing aesthetic patterns.

9. Economics:

• Profit Maximization: Parabolic functions are used to model cost, revenue, and profit functions in businesses to determine maximum or minimum points, which are crucial for decision-making.

10. Aerospace:

• Ballistic Missiles and Space Vehicles: The trajectory of rockets and missiles, especially before the introduction of guided systems, is essentially parabolic.

Q: What is an interesting fact about parabolas?

A: One particularly interesting fact about parabolas is their unique reflective property: a parabola reflects any incoming parallel beam of light (or other forms of waves such as sound waves) to a single point known as the focus. Conversely, a light source placed at the focus of a parabola will be reflected into a parallel beam along the axis of symmetry.

This property isn't just a mathematical curiosity; it has practical applications in various technologies and fields. For instance:

• Satellite dishes use this property to collect satellite signals, which come from a great distance and therefore essentially parallel, focusing them onto the receiver located at the focus.
• Automotive headlights and flashlights employ parabolic mirrors to direct light from a small bulb at the focus into a parallel beam, illuminating the road or path ahead effectively.
• Parabolic microphones gather sound waves into a focal point to amplify distant sounds, making them invaluable in nature recording, surveillance, and even in sports broadcasting to pick up distant sounds from the playfield.

The reflective property of parabolas presents a perfect blend of geometric beauty and practical utility, making parabolas a fascinating subject of study in mathematics and physics, with applications that touch many aspects of daily life and advanced technology.